 You hear about evolution
frequently in the media today. Recent court cases about teaching
of evolution and intelligent design keep the topic in the
spotlight. Through your coursework and discussions, you are
beginning to understand the basic components of the process of
evolution. Most students, when asked about evidence for
evolution, are aware of fossils and genetic studies on mutations.
Yet, have you ever wondered if there is a scientific way to determine
whether or not a particular trait is evolving? The answer is
yes. You hear about evolution
frequently in the media today. Recent court cases about teaching
of evolution and intelligent design keep the topic in the
spotlight. Through your coursework and discussions, you are
beginning to understand the basic components of the process of
evolution. Most students, when asked about evidence for
evolution, are aware of fossils and genetic studies on mutations.
Yet, have you ever wondered if there is a scientific way to determine
whether or not a particular trait is evolving? The answer is
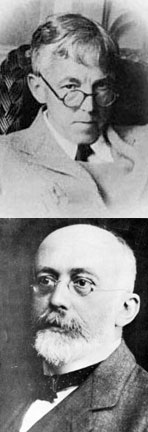
yes. Evolution can be defined as the change in allele frequencies (i.e., genotypes) over time. In the early 1900s, G. Hardy and W. Weinberg developed a mathematical model that predicts genotype frequencies when a population is not being affected by evolutionary forces. The model is known as the Hardy Weinberg Equilibrium Model. The Hardy-Weinberg equilibrium is a baseline against which the evolution of populations can be measured and is the foundation for the genetic theory of evolution. When a population is in Hardy-Weinberg equilibrium, there is no change in gene frequencies and, therefor, no evolution of the population. The conditions required for Hardy-Weinberg equilibrium are almost never met in nature, however. As a result, gene frequencies change and evolution occurs. If a population is not at Hardy-Weinberg equilibrium, then we know that one or more of the conditions required for the equilibrium are not being met. 
For the next two weeks, you will study how various factors affect gene frequencies of populations using a computer simulation. The simulations involve many of the concepts and terms that you already worked with and read about. You will see how genotypes change as a result of environmental pressures, population characteristics such as size, and chance effects. While the activity will demonstrate the process of changing organisms over time in a few minutes, it accurately models the actual process of evolution that occurs over decades to millions of years. Your Instructor will provide you with directions for using the computer simulations and for completing any written work that you need to turn in. |